If we assume that the reel is concentrated, all the conductors are situated at 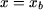 . Ampère's theorem applied to the contour which traverses the air-gap without containing the reel (contour 1 from figure 6, which traverses the air-gap at . Ampère's theorem applied to the contour which traverses the air-gap without containing the reel (contour 1 from figure 6, which traverses the air-gap at 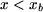 ) leads to: ) leads to:
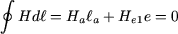 |
(21) |
Ampère's theorem applied to the contour which traverses the air-gap containing the reel (contour 2 from figure 6, which traverses the air-gap at 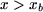 ) leads to: ) leads to:
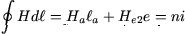 |
(22) |
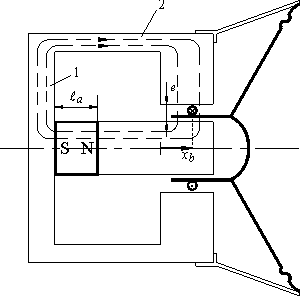
Figure 6
To obtain the 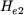 value we must, just as in the case of the calculation of the field produced by the permanent magnet, consider the conservation of the flux: value we must, just as in the case of the calculation of the field produced by the permanent magnet, consider the conservation of the flux:
- the flux which traverses the air-gap is:
- the flux which traverses the magnet is:
how to obtain 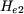 results: results:
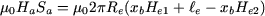 |
(23) |
From (21), (22) and (23) equations, we can deduce the value of 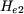 field: field:
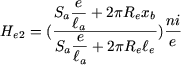 |
(24) |
The flux which traverses the reel is  times the flux which traverses the air-gap between times the flux which traverses the air-gap between 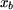 and and 
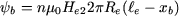 |
(25) |
Considering the (24) the result is
 |
(26) |
the 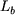 inductance of the reel is, therefore: inductance of the reel is, therefore:
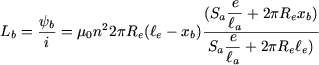 |
(27) |
the reluctant type of force which is exerted upon the reel will be:
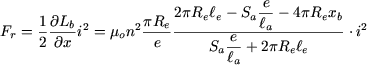 |
(28) |
This force which determines the presence of an unlineary term in the relation that connects the total force exerted upon the reel and the current which traverses it, can be reduced down to a negligible value comparing to the electrodynamic force, through an adequate dimensioning of the device. Generally,on this account, we only consider the electrodynamic term.
|